 Comics about mathematics, science, and the student life.
Comics about mathematics, science, and the student life.
Breakthrough
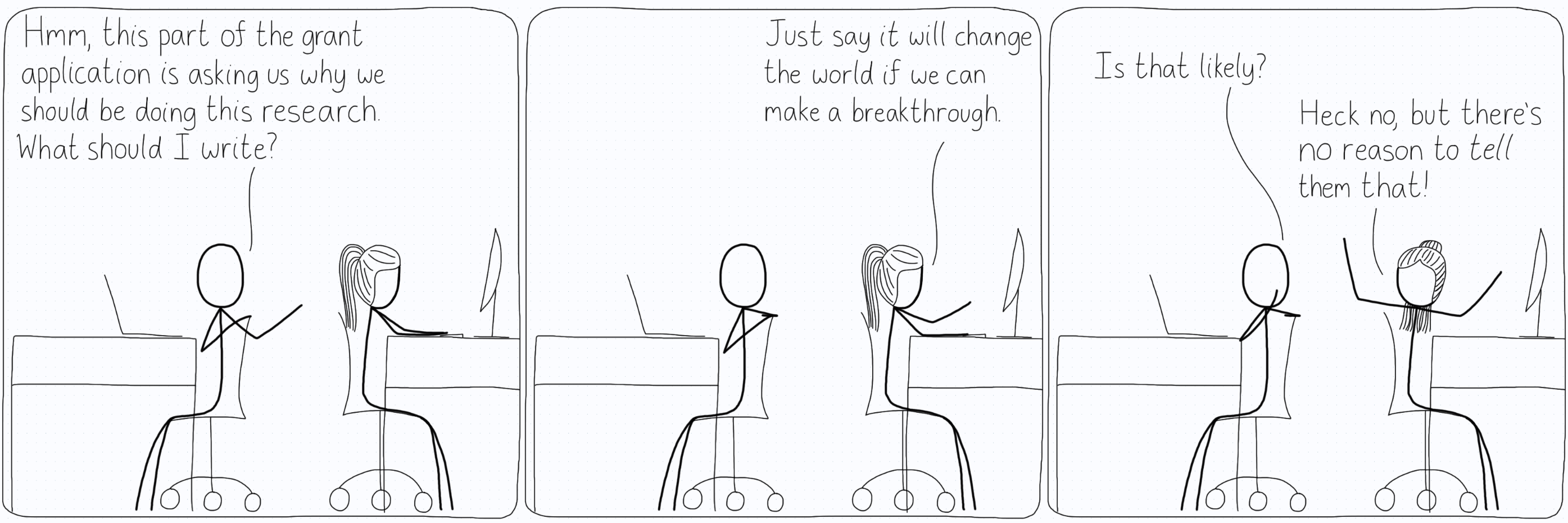
Ah, there’s nothing more relaxing than writing grant applications. They really make you indulge in your biggest fantasies for your research.
15 Jun 2020Basics

I sometimes notice myself rolling my eyes when I see another explanation of a basic concept that I know well, but then I realize that the target audience is not me.
Communicating to the public is important and distinct from communicating with experts.
08 Jun 2020Existential Risk

“Oh yeah, and that’s a good thing you won’t be able to sleep. That’s what I call ‘taking it seriously’!”
Disclaimer: I do not know what kind of values for such an app would be typical, but I think any number other than zero is a little terrifying.
03 Jun 2020Blending

I am only able to spot this within my own field, but I am certain that I get misled by experts in other fields when they feel compelled to mix in their own ideas.
There is no harm with stating your viewpoint, but it should be clearly distinct from the rest of the science. In that sense, I like thinking of good science communication like a layer cake. Each part is clearly marked so confusion is left to a minimum.
01 Jun 2020Academic Ghost Story

Bad notation will literally haunt you for weeks when you need to translate it in order to compare to your own work.
29 May 2020Positive

If you want to confuse a physics student, set a constant to be negative in a differential equation that would be harmonic motion. Chances are, they will zoom on and solve the problem without realizing their error.
27 May 2020Sorting

I prefer to think of it as simply letting my desk settle into its most natural state: pure chaos.
25 May 2020

